Parametric Equations
Practice
practice problem 1
solution
Start your solution by listing the given quantities along each axis and the time in convenient units…
| x0 = | 0 m |
| vx0 = | 0.050(3.00 × 108 m/s) |
| vx0 = | 1.50 × 107 m/s |
| ax = | 0 m/s2 |
| y0 = | 0 m |
| vy0 = | 0 m/s |
| ay = | −2.34 m/s2 |
| t = | 137 × 24 × 60 × 60 s |
| t = | 1.184 × 107 s |
Apply the one-dimensional equations of motion for constant acceleration in each direction to get the components of the displacement…
| x = | x0 + vx0Δt + ½axΔt2 |
| x = | vx0Δt |
| x = | (1.50 × 107 m/s)(1.184 × 107 s) |
| x = | +1.776 × 1014 m |
| y = | y0 + vy0Δt + ½ayΔt2 |
| y = | ½ayΔt2 |
| y = | ½(−2.34 m/s2)(1.184 × 107 s)2 |
| y = | −1.639 × 1014 m |
and velocity…
| vx = | vx0 + axΔt |
| vx = | vx0 |
| vx = | 1.5 × 107 m/s |
| vy = | vy0 + ayΔt |
| vy = | ayΔt |
| vy = | (−2.34 m/s2)(1.184 × 107 s) |
| vy = | −2.770 × 107 m/s |
Add the components to get the resultant displacement…
| r = | √[ x2 + y2 ] | |
| r = | √[(+1.776 × 1014 m)2 + (−1.639 × 1014 m)2] |
|
| r = | 2.42 × 1014 m | |
| tan θ = |
|
|||
| tan θ = |
|
|||
| θ = | −42.7° | |||
r = 2.42 × 1014 m at −42.7°
and the resultant velocity…
| v = | √[ vx2 + vy2 ] | |
| v = | √[(1.5 × 107 m/s)2 + (−2.770 × 107 m/s)2] |
|
| v = | 3.15 × 107 m/s | |
| tan θ = |
|
|||
| tan θ = |
|
|||
| θ = | −61.6° | |||
v = 3.15 × 107 m/s at −61.6°
practice problem 2
vx = 0.4 − 1.0 cos(t/100)
vy = 0.0 + 1.0 sin(t/100)
- the displacement as a function of time
- the path of the ship for the first 2000 s
- the direction of the ship at the beginning and end of this interval
- the maximum and minimum speed of the ship
solution
This is a problem that requires calculus. By definition, velocity is the first derivative of displacement with respect to time. From the fundamental theorem of calculus then, displacement is the time integral of velocity.
x = ∫ vx dt x = ∫(0.4 − 1.0 cos(t/100)) dt x = 0.4 t − 100 sin(t/100) + cx y = ∫ vy dt y = ∫(0.0 + 1.0 sin(t/100)) dt y = − 100 cos(t/100) + cy All that remains is to adjust the constants for the initial conditions…
x0, y0 = 0
like this…
0 = 0.4(0) − 100 sin(0/100) + cx 0 = cx cx = 0 0 = −100 cos(0/100) + cy 0 = −100 + cy cy = 100 Therefore…
x = 0.4 t − 100 sin(t/100) y = 100 − 100 cos(t/100) The ship is moving forward in the x direction, as the first term shows, but also oscillating, as the second term shows. The coefficient of the second term is much larger than the first, so we can be quite certain that there will be times when the ship will reverse direction, but overall the motion will be forward. It's sort of a "two steps forward, one step back" kind of kind of shuffle.
At the same time, the ship is oscillating in the y direction. The constant term is basically unimportant. The ship starts at a particular location and then dodges side to side without ever making any headway in this direction.
Combining these two motions results in a path that looks something like a doodle from a bored physics student's notebook. Don't try to sketch it. Let a computer or graphing calculator draw it for you.
To determine the direction of motion, evaluate the velocity equations at the appropriate times.
vx(t) = 0.4 − cos(t/100) vx(0) = 0.4 − cos(0/100) vx(0) = 0.4 − 1.0 vy(t) = 0.0 + sin(t/100) vy(0) = 0.0 + sin(0/100) vy(0) = 0.0 + 0.0 At the beginning of the interval, the velocity was entirely in the negative x direction.
vx(t) = 0.4 − cos(t/100) vx(2000) = 0.4 − cos(2000/100) vx(2000) = 0.4 − 0.4 vy(t) = 0.0 + sin(t/100) vy(2000) = 0.0 + sin(2000/100) vy(2000) = 0.0 + 0.9 At the end of the interval, the velocity was entirely in the positive y direction.
Speed is the magnitude of velocity and is found by adding the components using the Pythagorean theorem.
v2 = vx2 + vy2 v2 = (0.4 − cos(t/100))2 + (0.0 + sin(t/100))2 v2 = 0.16 − 0.8 cos(t/100) + cos2 (t/100) + sin2 (t/100) v2 = 0.16 − 0.8 cos(t/100) + 1.00 v2 = 1.16 − 0.8 cos(t/100) The cosine term is what matters here. It has its maximum value of +1 when t/100 is 0, 2π, 4π and other even multiples of π and its minimum value of −1 when t/100 is π, 3π, 5π and other odd multiples of π. To determine the range of the ship's speed, simply substitute ±1 for the cosine term.
v2 = 1.16 − 0.8 cos(t/100)
vmin = √(1.16 −1.00) vmin = √0.16 vmax = √(1.16 +1.00) vmax = √2.16
practice problem 3
| x = A sin (at + φ) | ||
| y = B sin (bt) | ||
| x, y = | coordinates on a plane |
| t = | parameter |
| A, B = | amplitudes |
| a, b = | angular frequencies |
| φ = | phase angle |
| −1.5 < x < +1.5 | ||
| −1.5 < y < +1.5 | ||
- Set the parameter range to one lap around the unit circle (0 < t < 2π).
- Set the angular frequencies to one (a = b = 1). Draw the lissajous figures for various phase angles φ (e.g.; 0, π/4, π/2, π, …). What effect does this have on the lissajous figure?
- Set the phase angle equal to zero (φ = 0). Draw the lissajous figures for various whole number angular frequencies a and b (e.g.; 1 and 2, 2 and 5, 3 and 8, …). What effect does this have on the lissajous figure?
- Set the parameter range to one hundred laps around the unit circle (0 < t < 200π) or some other large number. Leave the phase angle set to zero (φ = 0).
- Set one of the frequencies equal to 1 and the other equal to an irrational number like √2, π, or e. What effect does this have on the lissajous figure?
solution
Results…
When the two frequencies are equal, you basically get an ellipse. Changing the phase angle changes the eccentricity — from very squashed on a 45° axis, to perfectly circular, to very squashed on a 135° axis, to perfectly circular, and back.
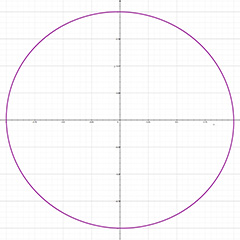
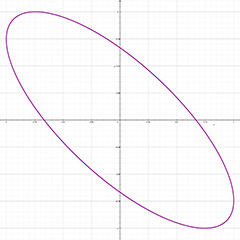
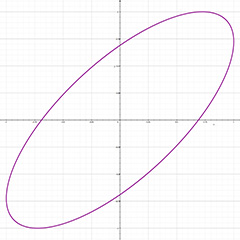
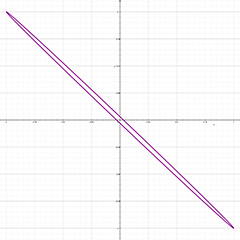
(animate) 
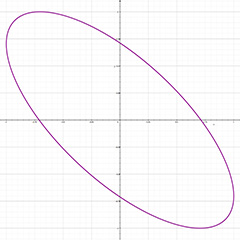
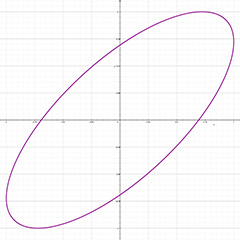
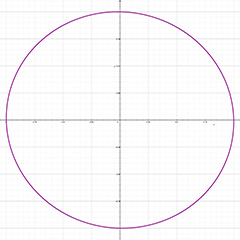
Whole number frequencies form curves that look like braided strings. When at least one frequency is an even number, the curves are closed (animate).
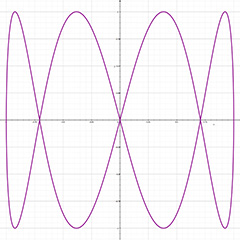
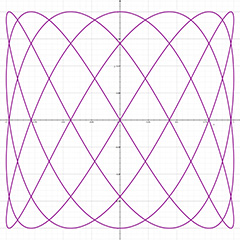
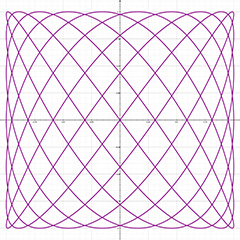

When both frequencies are odd numbers, the curve oscillates back and forth between two end points (animate).
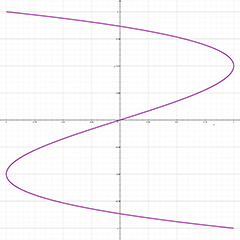
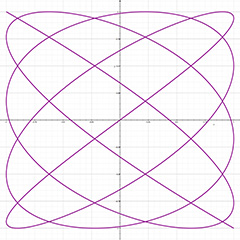
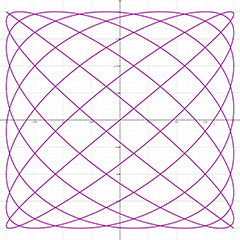
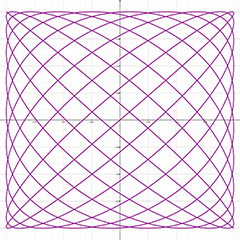
When the ratio of the two frequencies is irrational, the curve never retraces itself and never terminates. It will pass through every point of its domain after an infinite amount of parameter time has passed. A curve like this is said to be space filling (animate).
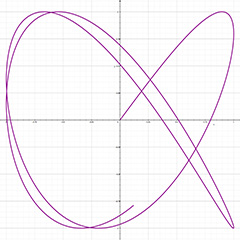
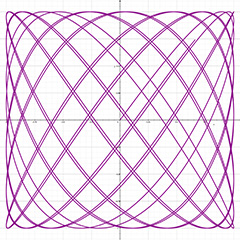


practice problem 4
solution
Answer it.
